ЭФФЕКТ ЧАСТИЧНО-ДЫРОЧНОГО ВЗАИМОДЕЙСТВИЯ ДЛЯ ЭНЕРГИИ ОТДЕЛЕНИЯ ПРОТОНОВ И НЕЙТРОНОВ В ЗЕРКАЛЬНЫХ ЯДРАХ, БЛИЗКИХ К СИММЕТРИЧНЫМ

Ершов Д. К.

Кандидат физико-математических наук, Смоленский государственный университет

ЭФФЕКТ ЧАСТИЧНО-ДЫРОЧНОГО ВЗАИМОДЕЙСТВИЯ ДЛЯ ЭНЕРГИИ ОТДЕЛЕНИЯ ПРОТОНОВ И НЕЙТРОНОВ В ЗЕРКАЛЬНЫХ ЯДРАХ, БЛИЗКИХ К СИММЕТРИЧНЫМ

Аннотация

Используя экспериментальные данные рассчитаны энергия отделения протонов и нейтронов в зеркальных ядрах с Z=N±1. Энергии отделения из нечетных подоболочек больше, чем из четных. После Z≥32 эта аномалия исчезает.

Ключевые слова: зеркальные ядра, протоны, нейтроны, энергии отделения, частично-дырочное взаимодействие.

Ershov D. K.

Candidate of Physical and Mathematical Sciences, Smolensk State University.

THE EFFECT OF PARTICLE-HOLE INTERACTION TO THE ENERGY SEPARATION OF PROTONS AND NEUTRONS IN FAMILIES MIRROR NUCLEI AT NEARLY SYMMETRICAL

Abstract

By the used of experimental date are calculated an energy separation for the protons and neutrons in the family mirror nuclei with Z=N±1. Of the energy separation from nuclei with odd nucleon subshell is the more then with even subshell. This anomaly vanish after Z≥32.

Keywords: mirror nucleus, protons, neutrons, energy branch, particle-hole interaction.

1. Introduction

At present time, there are no unified microscopic theory, which from first principles explains all the properties of atomic nuclei. [1, 2]

This is largely due to the fact that the atomic nuclei even medium-sized, and even more heavy elements, are multiparticle systems consisting of strongly interacting nucleons. Therefore, for the study of nuclear properties, processes of nuclear interactions has to use different semiempirical approaches appropriate to different model representations (mean-field theory, shell model, model of pair interactions). It should be noted that the finite of the sizes of nuclei leads to additional difficulties in their theoretical description. Infinite system turns out to be easier to describe. A special place among the famous atomic nuclei occupy so-called mirror nuclei. This pair of nuclei which the number of neutrons in one equals the number of protons in another N₁=Z₁ and conversely N₂=Z₁.

In such nuclei in the most refined can explore some nuclear effects - for example, energy symmetry which ~(N-Z)².

In this paper calculates the energy separation of protons S_p and neutrons S_n in families mirror nuclei with Z=N±1.

2. Methodology

Energy separation are determined by the known method

S_p(Z,N)=M(Z-1,N)+M_H-M(Z,N)                                    (1)

S_p(Z,N)=M(Z,N-1)+M_H-M(Z,N)                                    (2)

Here M is the mass of the relevant isotopes, M_n - the mass of a neutron, M_H - mass of hydrogen atoms. Often, instead of a mass of use value (M-A). The final results for S_p and S_n, is not change as a consequence of the conservation of baryon charge (in this case A).

If you use the available experimental data [3, 4] for the corresponding mirror nuclei up Z=40, you can calculate the energy separation S_p and S_n.

3. Results and Discussion

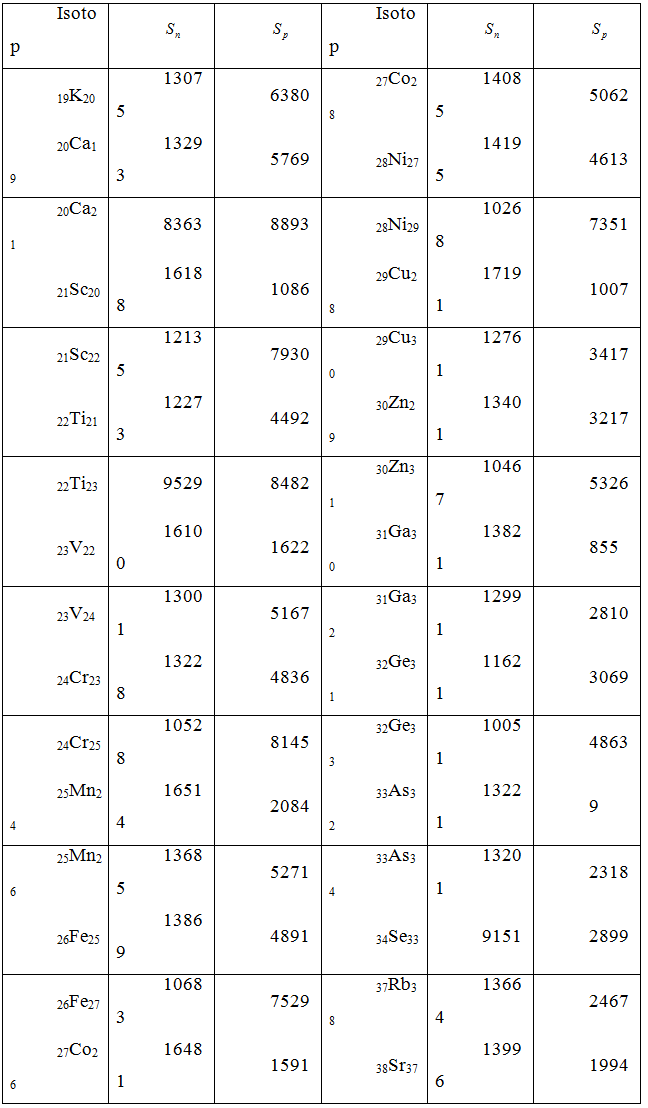
The results of calculations are presented in the table.

Table 1 - Energy separation (in Kev).

Calculations were done for Z=12 ÷ 40, but in order to save we give the values only for the most interesting area of values Z.

The results of calculations shows that for some couples mirror nuclei is observed anomaly: energy separation S_p and S_n  for odd subhell exceed the corresponding value for even subhell. After Z=32 this anomaly disappears (except for a pair of  and ). It is easy to notice, that all «normal» pair in the model  clustering can be presented as mα+₁H₀ or mα+₀n₁, and all abnormal pairs in the form mα+₁H₂ or mα+₂He₁, m- the number of α particles (₂He₂).

The fact that the energy separation of a single of the valence neutrons and protons is less than the energy separation of nucleons from closed subhell ₂He₂ quite understandable. But why energy  separation  of protons from ₂He₁ less energy separation of proton from ₁H₂, and energy separation of neutrons from ₁H₂ less energy separation of protons from ₂He₁ not entirely clear. Of course, the shell model are its sphere application and the nucleons are moving in a self-consistent field all other nucleons, but the effects pairing of identical nucleons in nuclei have long been considered established.

You should pay attention to the fact that the anomaly disappears after Z=32. In this area, the binding energy of nucleons reach maximum (nuclear saturation).

The biggest specific binding energy of the nucleus ₂₈Ni₂₈, but this nucleus is specific - it doubly magic. In the recent paper [5] it is stated that the correlation effects are maximized at Z=32.

It is also interesting to note that  and  may be regarded as a respectively with proton and neutron holes. Particle-hole interaction actively used in various variants of modern microscopic theory of the nucleus [1, 2], and the anomaly may reflect the changing nature of the interaction of the particle-hole state in the region Z=32 in the mirror nuclei.

Quartet (actually α partial) clustering symmetric (Z=N) nuclei in was considered in [6]. There was studied isovector pairing - alpha-particle presented as a system of two isovector pairs.

It should be noted that in the vicinity of the magical Z=28 no radical changes are taking place. These changes are observed at Z≥32 when filling starts proton 1f_5/2 subshell. It ends with Z=38, and then begins the collectivization filled (intruders) configuration of high orbits. It leads, for example, to the absence of closed subshell when Z=40, Z=40, [7]. Perhaps the single return anomalies for ₃₇Rb₃₈ and ₃₈Sr₃₇ related to the end of filling subshell.

4.Conclusion

In conclusion, we note that, perhaps for the first time showed the influence of the particle-hole interaction to the energy separation of the nucleons in the mirror nuclei. It is interesting to investigate and other characteristics of these mirrored pairs on the influence of these anomalies.

Литература

1. МигдалА. Б. Теория конечных Ферми-систем и свойства атомных ядер. М.: Наука, 1983.
2. СоловьевВ. Г. Теория атомных ядер – квазичастицы и фононы. М.: Энергоатомиздат, 1989.
3. Физические величины. Справочник. М.: Энергоатомиздат, 1991.
4. Атомные массы, 2011.
5. Porquet M.-G. and Sorlin O. Phys. Rev. C85.014303 (2012)
6. Sanduliscu N., Neqrea D., Dukelsky J. and Johnson C.W. Phys. Rev. C85.061303 (2012)
7. Naimi S. G. Audi, Beek D., Blaum K. et all. Phys Rev. C85.014325 (2012)

References

1. Migdal A. B. Teorija konechnyh Fermi-sistem i svojstva atomnyh jader. M.: Nauka, 1983.
2. Solov'ev V. G. Teorija atomnyh jader – kvazichasticy i fonony. M.: Jenergoatomizdat, 1989.
3. Fizicheskie velichiny. Spravochnik. M.: Jenergoatomizdat, 1991.
4. Atomnye massy, 2011.
5. Porquet M.-G. and Sorlin O. Phys. Rev. C85.014303 (2012)
6. Sanduliscu N., Neqrea D., Dukelsky J. and Johnson C.W. Phys. Rev. C85.061303 (2012)
7. Naimi S. G. Audi, Beek D., Blaum K. et all. Phys Rev. C85.014325 (2012)