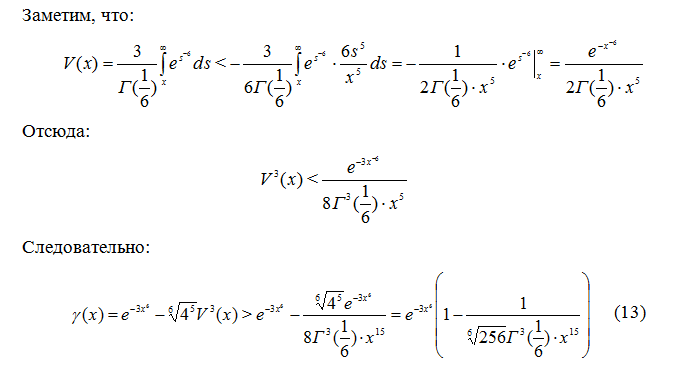ОБ ОДНОЙ ИНТЕГРАЛЬНОЙ ОЦЕНКЕ

Пучнин Р.В.¹, Швец Ю.В.², Миллер Н.В.³

¹ Кандидат технических наук, ² Кандидат педагогических наук, ³ Кандидат педагогических наук, Сибирский государственный университет путей сообщения

ОБ ОДНОЙ ИНТЕГРАЛЬНОЙ ОЦЕНКЕ

Аннотация

 

Ключевые слова: интегральные неравенства, гамма-функция, степенные оценки, несобственный интеграл, логарифмически выпуклая функция.

 

Puchnin R.V.¹, Shvets Yu.V.², Miller N.V.³

¹PhD in Engineering, ²PhD in Pedagogy, ³PhD in Pedagogy, Siberian State Transport University

ABOUT ONE INTEGRATED ASSESSMENT

Abstract

  Keywords: integrated inequalities, gamma function, sedate estimates, not own integral, logarithmic convex function.  

Введение

Полученная степенная оценка может быть использована в статистике при получении точечных и интервальных оценок неизвестных параметров распределений, а также в эконометрике при анализе остатков трендовых моделей с полиномиальной структурой лага.

Кроме того, справедливо следующее утверждение.

Теорема 1.2. Оценка в теореме 1.1 остается справедливой при 0 ≤ k <1 для любого положительного х.

Доказательство теоремы 1.2

Теорема 1.2 доказана.

Доказательство основного результата

Доказательство теоремы 1.1 проводятся по схеме, близкой к рассмотренной в работах [5], [7].

При k = 1 оценка (2) вытекает из того, что для любого действительного х справедливо неравенство 0 < V(x) < 1.

Теорема 1.1 доказана.

Литература

1. Н. Alzer. On some inequalities for the incomplete gamma function, Math. Comp. 66 (1997), no 218, 771-778
2. P. Natalini and B. Palumbo. Inequalities for the incomplete gamma function, Math. Inequal. Appl. 3 (2000), no. 1, 69-77
3. F. Qi, Monotonicity results and inequalities for the gamma and incomplete gamma functions, Math. Inequal. Appl. 5 (2002), no. 1, 61-77
4. A. Baricz. A functional inequality for the survival function of the Gamma distribution, J. Inewual. Pure and Appl. Math., 9, 1 (2008), Article 13.
5. Xiao-Li Hu. Two new inequalities for Gaussian and gamma distributions, Journal of mathematical inequalities. Volume 4, Number 4 (2010), 609-613
6. Пекельник H.M., Хаустова О.И., Трефилова И.А. Замечания об одном интегральном неравенстве. X международная научно-практическая конференция: «Научные перспективы XXI века. Достижения и перспективы нового столетия» №3(10) 2015, часть 9, 72 -74
7. Пожидаев А.В., Пекельник Н.М., Хаустова О.И., Трефилова И.А. Об оценке четных степеней срезок некоторых интегралов, Наука и мир Международный научный журнал, №1 (17), 2015, том 1, 29 – 34

References

1. Н. Alzer. On some inequalities for the incomplete gamma function, Math. Comp. 66 (1997), no 218, 771-778
2. P. Natalini and B. Palumbo. Inequalities for the incomplete gamma function, Math. Inequal. Appl. 3 (2000), no. 1, 69-77
3. F. Qi, Monotonicity results and inequalities for the gamma and incomplete gamma functions, Math. Inequal. Appl. 5 (2002), no. 1, 61-77
4. A. Baricz. A functional inequality for the survival function of the Gamma distribution, J. Inewual. Pure and Appl. Math., 9, 1 (2008), Article 13.
5. Xiao-Li Hu. Two new inequalities for Gaussian and gamma distributions, Journal of mathematical inequalities. Volume 4, Number 4 (2010), 609-613
6. Pekelnik H.M., Haustova O. I., Trefilova I.A. Remarks on one integrated inequality. X international scientific and practical conference: "Scientific prospects of HHI of an eyelid. Achievements and prospects of new century" No. 3(10) 2015, part 9, 72-74
7. Pozhidayev A.V., Pekelnik N. M., Haustova O. I., Trefilova I.A. About an assessment of even extents of cuts of some integrals, Science and the world the International scientific magazine, No. 1 (17), 2015, volume 1, 29 - 34