NON-LINEAR DIFFERENTIAL EQUATIONS OF HIGHER ORDER

Перов А. И.¹, Каверина В.К.²

¹Доктор физико-математических наук, Воронежский государственный университет, ²Кандидат физико-математических наук, Воронежский государственный архитектурно-строительный университет

Работа выполнена при поддержке гранта РФФИ №16-01-00197

НЕЛИНЕЙНЫЕ ДИФФЕРЕНЦИАЛЬНЫЕ УРАВНЕНИЯ ВЫСШИХ ПОРЯДКОВ

Аннотация

В статье изучается вопрос существования периодических решений у возмущенного дифференциального уравнения -го порядка. Полученные теоремы навеяны проблемой В.И. Зубова и связаны с понятиями асимптотической устойчивости в целом и устойчивости по Дирихле.  Доказательства этих теорем носят топологический характер и опираются на понятие степени отображения.

Ключевые слова: нелинейные скалярные дифференциальные уравнения высшего порядка, периодические решения (свободные и вынужденные колебания), топологическая степень отображения, метод направляющих функций.

Perov A. I.¹, Kaverina V. K.²

¹PhD in Physics and Mathematics, Voronezh State University,  ²PhD in Physics and Mathematics, Voronezh State University of Architecture and Civil Engineering

NON-LINEAR DIFFERENTIAL EQUATIONS OF HIGHER ORDER

Abstract

In this article we study the problem of the existence of periodic solutions of the perturbed  differential equations of higher order. These theorems were inspired by the work of V.I. Zubov and associated with such notions as asymptotically stability in the large and stability in the Dirichlet's sense in the large. Proofs of these theorems have a topological sense.

Keywords: nonlinear scalar differential equations of higher order, periodic solutions (free and forced oscillations), topological degree of mapping, method of guiding functions.

 

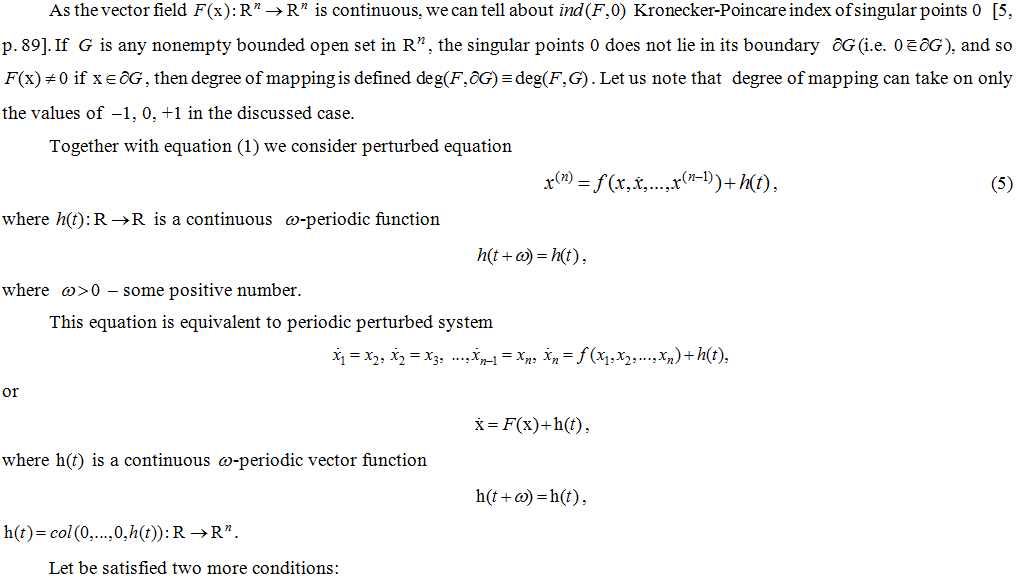
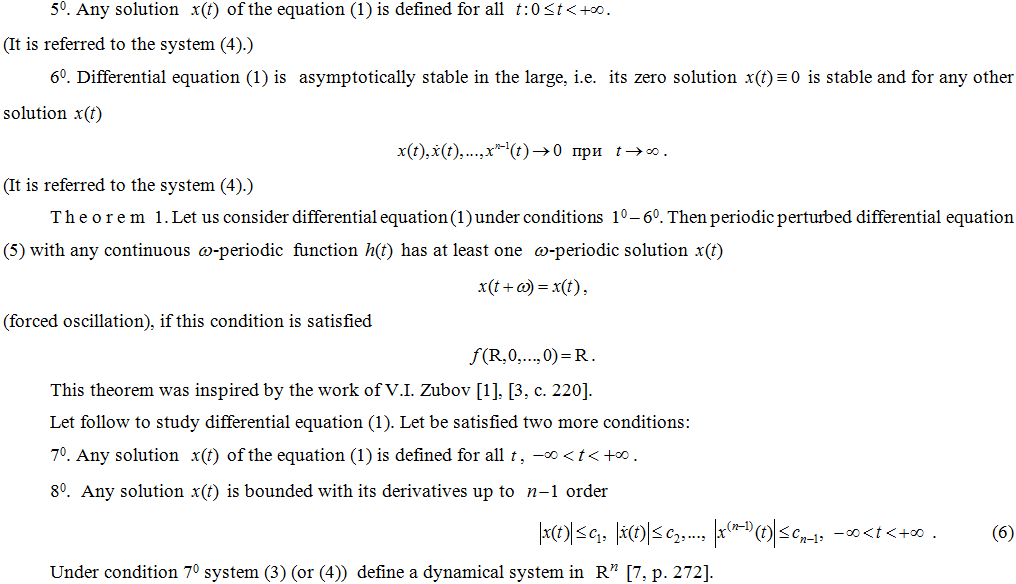
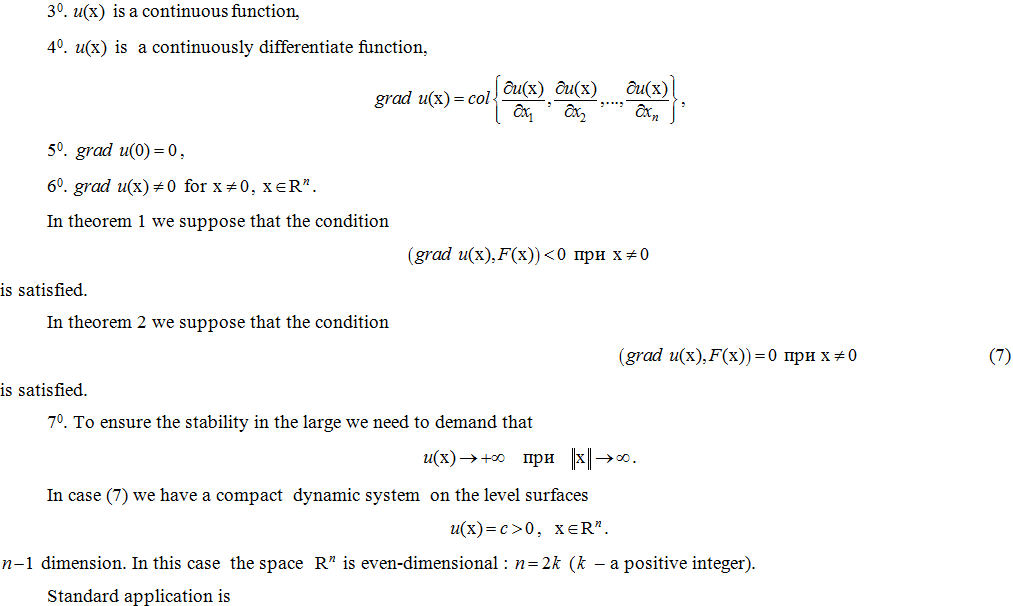
References

1. Evchenko V.К. About one problem from the theory of oscillations // Tambov University Reports. – 2015. – Volume 20, issue 5. – P. 1136-1137.
2. Borovskikh A.V., Perov А.I. Lectures on the ordinary differential equations. Moscow-Izhevsk: Regular and Chaotic Dynamics, 2004. – 540 p.
3. Zubov V.I. The theory of oscillations. М.: “Vusshaya Shkola”, 1979. – 400 p.
4. Krasnosel'skii М.А., Perov А.I. About one principle of the existence of the periodic and almost periodic solutions of the system of ordinary differential equations. DAN USSR, 1958. – Volume 128, №2. – P. 235-238.
5. Krasnosel'skii М.А. The shift operator on the path of the differential equations. М.: Nauka, 1960. – 332 p.
6. Krukov B.I. Forced oscillations of the essentially nonlinear system. М.: Mechanical Engineering, 1984. – 216 p.
7. Petrovskii I.G. Lectures on the ordinary differential equations. М.: Nauka, 1964. – 272 p.
8. Perov А.I. Some questions of the qualitative theory of the differential equations. Cand. of phys.-math. scien. thesis – Voronezh, 1959. – 123 p.
9. Perov А.I. York theorem and Wirtinger inequality // Mathematical Notes, 2001. – Volume 70, issue 2. – P. 237-245.
10. Perov А.I., Evchenko V.К. Method of guiding functions. Voronezh: Publisher's printed center VGU, 2012. – 182 p.
11. Reissig R., Sansone G., Conti R. Non-linear differential equations of higher order. Leyden: Noordhoff International Publishing, 1974. – 670 p.