ASYMPTOTICS OF THE EIGENVALUES OF A FOURTH-ORDER FUNCTIONAL-DIFFERENTIAL OPERATOR WITH SUMMABLE POTENTIAL
АСИМПТОТИКА СОБСТВЕННЫХ ЗНАЧЕНИЙ ОДНОГО ФУНКЦИОНАЛЬНО-ДИФФЕРЕНЦИАЛЬНОГО ОПЕРАТОРА ЧЕТВЁРТОГО ПОРЯДКА С СУММИРУЕМЫМ ПОТЕНЦИАЛОМ
Научная статья
Митрохин С.И. *
Московский государственный университет им. М. В. Ломоносова, Москва, Россия
* Корреспондирующий автор (mitrokhin-sergeyх[at]yandex.ru)
АннотацияВ статье изучаются спектральные свойства функционально-дифференциального оператора четвёртого порядка с суммируемым потенциалом. Граничные условия являются разделёнными. Решение функционально-дифференциального уравнения сведено к решению интегрального уравнения Вольтерра. При больших значениях спектрального параметра выведена асимптотика решений функционально-дифференциального уравнения, задающего исследуемый оператор. При помощи найденной асимптотики решений изучены граничные условия оператора. Получено уравнение на собственные значения изучаемого оператора. Найдена асимптотика собственных значений исследуемого функционально-дифференциального оператора.
Ключевые слова: спектральная теория, функционально-дифференциальный оператор, спектральный параметр, суммируемый потенциал, асимптотика решений, асимптотика собственных значений, собственные функции.
ASYMPTOTICS OF THE EIGENVALUES OF A FOURTH-ORDER FUNCTIONAL-DIFFERENTIAL OPERATOR WITH SUMMABLE POTENTIAL
Research article
Mitrokhin S.I. *
Lomonosov Moscow State University, Moscow, Russia
* Corresponding author (mitrokhin-sergeyх[at]yandex.ru)
AbstractIn this paper the spectral properties of a fourth-order functional-differential operator with a summable potential are studied. The boundary conditions are separated. The solution of the functional-differential equation is reduced to the solution of the Volterra integral equation. For large values of the spectral parameter, the asymptotics of the solutions of the functional-differential equation that defines the investigated operator is derived. Using the found asymptotics of solutions, the boundary conditions of the operator are studied. The equation for the eigenvalues of the operator is obtained. The asymptotics of the eigenvalues of the functional-differential operator under investigation is found.
Keywords: spectral theory, functional-differential operator, spectral parameter, summable potential, asymptotics of solutions, asymptotics of eigenvalues, eigenfunctions.
IntroductionConsider a functional-differential operator given by a fourth-order differential equation
![]() (1)
(1)
with boundary conditions of the form
![]() (2)
(2)
under the assumption of the summability of the potential ![]() :
:
![]() (3)
(3)
almost everywhere on the segment ![]() .
.
We assume that in equation (1) the weight function ![]() is constant:
is constant: ![]() and the delay value is a smooth and increasing function: there is a derivative
and the delay value is a smooth and increasing function: there is a derivative ![]() .
.
Historical review
Differential operators with nonsmooth coefficients began to be studied not so long ago. In the classical paper [1], for the second-order differential operator, the convergence of expansions in eigenfunctions at the points of discontinuity of the coefficients was studied.
In the article [2] the differentiation operators of the first and second order with a discontinuous alternating weight function were studied. Regularized traces for the functional-differential operator of the second order were calculated by the author in [3]. The spectral properties of second-order operators with a discontinuous weight function were studied in [4]. In article [5] the second-order operators with summable potential were first studied. The method of article [5] does not carry over to operators of order higher than the second.
In the article [6] the author demonstrated a method for studying the spectral properties of a fourth-order operator with a summable potential. The Sturm-Liouville operators with singular coefficients were studied in [7]. A sixth-order differential operator with summable coefficients with a retarded argument was studied by the author in [8].
In article [9] the regularized trace of a second-order operator whose potential is a Delta function was calculated. Differential operators of odd order with multipoint boundary conditions with summable potential were studied by the author in [10]. Operators of the form (1) - (2) - (3) have not been studied by anyone before.
Asymptotics of solutions of differential equation (1)
Let ![]() , moreover, that branch of the root for which
, moreover, that branch of the root for which ![]() is fixed. Let be the various roots of the fourth degree of unity:
is fixed. Let be the various roots of the fourth degree of unity:
![]() (4)
(4)
The following theorem is proved by the method of variation of arbitrary constants.
Theorem 1. The solution y(x,s) of the functional differential equation (1) is the solution of the following Volterra integral equation:
![]() (5)
(5)
 (6)
The last sum in equality (6) is zero by virtue of property (4). Similarly we have:
(6)
The last sum in equality (6) is zero by virtue of property (4). Similarly we have:
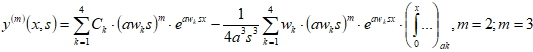 (7)
(7)
Let's differentiate the formula (7) by m=3 for the variable x, substitute the resulting expression and (5) in equation (1), we get the correct equality. So, indeed, the formula (5) of theorem 1 is correct.
Next, we apply the method of successive Picard approximations: find a function ![]() from the formula (5), substitute the resulting expression in (5), and get:
from the formula (5), substitute the resulting expression in (5), and get:
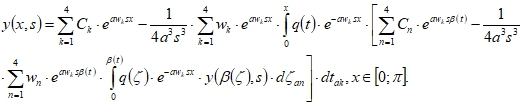 (8)
(8)
By opening the brackets in formula (8) and rearranging the terms, we obtain the following statement.
Theorem 2. The general solution of the functionally differentiable equation (1) has the following form:
![]() (9)
(9)
where ![]() are arbitrary constants, and for the fundamental system of solutions
are arbitrary constants, and for the fundamental system of solutions ![]() of equation (1) for
of equation (1) for ![]() the following asymptotic estimates are valid:
the following asymptotic estimates are valid:

Estimates of the form (10)-(11) are obtained similarly to the estimates of the monograph [11, chapter 2].
Study of boundary conditions (2). The presence of formulas (9)-(14) allows us to study the boundary conditions (2). From formula (9) we have:
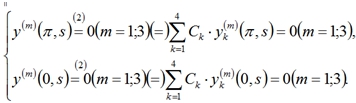 (15) (16)
(15) (16)
System (15) – (16) is a homogeneous system of four linear equations with four unknowns ![]() . It follows from Kramer's method that such a system has a nonzero solution
. It follows from Kramer's method that such a system has a nonzero solution ![]() only when its determinant is zero. Therefore, the following statement is true.
only when its determinant is zero. Therefore, the following statement is true.
Theorem 3. The equation for eigenvalue of the functional differential operator (1) – (2) – (3) has the following form:
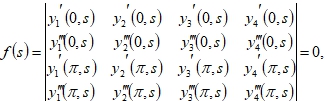 (17)
(17)
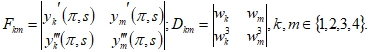 (19)
where the following notation is introduced:
(19)
where the following notation is introduced:
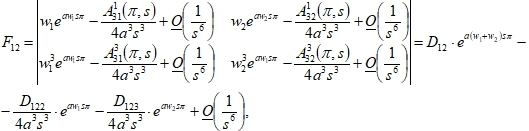 (23)
where the notation is introduced:
(23)
where the notation is introduced:
Similarly, it is possible to write out functions ![]() from (22).
from (22).
The asymptotics of the eigenvalues of the operator (1) - (2) - (3)
The equation (22) – (24) will be studied by the methods of work [3], [4]. The indicator diagram of this equation (see [12, chapter 12]) is a square ABCD, the coordinates of the vertices of this square are as follows: ![]() . From the general theory of finding the roots of equation (22) – (24) (see [12, chapter 12]), it follows that the roots are in four sectors of an infinitesimal angles, the bisectors of which are middle-perpendiculars to the sides of the square ABCD. Studying the roots of equation (22) - (24) using the methods of works [8], [10], we conclude that the following statement is true.
. From the general theory of finding the roots of equation (22) – (24) (see [12, chapter 12]), it follows that the roots are in four sectors of an infinitesimal angles, the bisectors of which are middle-perpendiculars to the sides of the square ABCD. Studying the roots of equation (22) - (24) using the methods of works [8], [10], we conclude that the following statement is true.
Theorem 3. Asymptotics of eigenvalues of the functional differential operator
(1) – (2) – (3) in the sector 2) (which corresponds to the segmentSubstituting the formulas (25) into equation (22) – (24), we apply the formulas
(12) – (13) and Taylor's formulas, we equate the coefficients at the same powers ![]() , we calculate the coefficient
, we calculate the coefficient ![]() from (25) in explicit form:
from (25) in explicit form:
 (26)
(26)
Using formulas (25) - (28), we can find the asymptotic behavior of the eigenfunctions of the operator (1) - (2) - (3) and solve the question of their completeness and basis property. The proposed method for studying functional differential operators can be transferred to sixth and eighth order operators with summable potential.
| Конфликт интересов Не указан. | Conflict of Interest None declared. |
Список литературы / References
- Ильин В. А. О сходимости разложений по собственным функциям в точках разрыва коэффициентов дифференциального оператора / В. А. Ильин // Математические заметки. — 1977. — Т. 22. — № 5. — С. 698-723.
- Гуревич А. П. Операторы дифференцирования первого и второго порядков со знакопеременной весовой функцией / А. П. Гуревич, А. П. Хромов // Математические заметки. — 1994. — Т. 56. — № 1. — С. 653-661.
- Митрохин С. И. О формулах следов для одной краевой задачи с функционально-дифференциальным уравнением с разрывным коэффициентом / С. И. Митрохин // Дифференциальные уравнения. — — Т. 22. — № 6. — С. 927-931.
- Митрохин С. И. О некоторых спектральных свойствах дифференциальных операторов второго порядка с разрывной весовой функцией / С. И. Митрохин // Доклады РАН. — — Т. 356. — № 1. — С. 13-15.
- Винокуров В. А. Асимптотика любого порядка собственных значений и собственных функций краевой задачи Штурма—Лиувилля на отрезке с суммируемым потенциалом / В. А. Винокуров, В. А. Садовничий // Известия РАН. Сер.: матем. — 2000. — Т. 64. — № 4. — С. 47-108.
- Митрохин С. И. Асимптотика собственных значений дифференциального оператора четвёртого порядка с суммируемыми коэффициентами / С. И. Митрохин // Вестник Московского университета. Сер.: матем., мех. — 2009. — № 3. — С. 14-17.
- Савчук А. М. Операторы Штурма-Лиувилля с сингулярными потенциалами / А. М. Савчук, А. А. Шкаликов // Математические заметки. — 1999. — Т. 66. — № 6. — С. 897-912.
- Митрохин С. И. О спектральных свойствах одного дифференциального оператора с суммируемыми коэффициентами с запаздывающим аргументом / С. И. Митрохин // Уфимский математический журнал. — 2011. — Т. 3. — №4. — С. 95-115.
- Савчук А. М. Регуляризованный след первого порядка оператора Штурма-Лиувилля с –потенциалом / А. М. Савчук // УМН. — — Т. 55. — №6 (336). — С. 155-156.
- Митрохин С. И. Об изучении спектра многоточечной краевой задачи для дифференциального оператора нечётного порядка с суммируемым потенциалом / С. И. Митрохин // Математические заметки СВФУ. — 2017. — Т. 24. — № 1. — С. 26-42.
- Наймарк М. А. Линейные дифференциальные операторы. / М. А. Наймарк // М.: Наука. — — 528 с.
- Беллман Р. Дифференциально-разностные уравнения. / Р. Беллман, К. Л. Кук // М.: Мир. 1967. — 548 с.
References in English / Список литературы на английском языке
- Il'in V. A. O skhodimosti razlozhenij po sobstvennym funkciyam v tochkah razryva koefficientov differencial'nogo operatora [On the convergence of expansions in eigenfunctions at points of discontinuity of the coefficients of a differential operator] / V.A. Il'in. // Matematicheskie zametki [Mathematical notes]. — 1977. — V. 22. — No. 5. — P. 698-723. [in Russian]
- Gurevich A. P. Operatory differencirovaniya pervogo i vtorogo poryadkov so znakoperemennoj vesovoj funkciej [Operators of differentiation first and second order with an alternating weight function] / A. P. Gurevich, A. P. Khromov // Matematicheskie zametki [Mathematical notes]. — 1994. — V. 56. — No. 1. — P. 3-15. // Math. Notes. – 1994. – Vol. 56, iss. 1, P. 653-661. [in Russian]
- Mitrokhin S. I. O formulah sledov dlya odnoj kraevoj zadachi s funkcional'no-differencial'nym uravneniem s razryvnym koefficientom [About formulas for traces for a boundary value problem with functional-differential equation with a discontinuous coefficient] / S. I. Mitrokhin // Differencial'nye uravneniya. [Differential equations]. — 1986. — V. 22. — No. 6. — P. 927-931. [in Russian]
- Mitrokhin S. I. O nekotoryh spektral'nyh svojstvah differencial'nyh operatorov vtorogo poryadka s razryvnoj vesovoj funkciej [On some spectral properties of differential operators of second order with discontinuous weight function] / S. I. Mitrokhin // Doklady RAN [Reports of the Russian Academy of Sciences]. — 1997. — V. 356. — No. 1. — P. 13-15. [in Russian]
- Vinokurov V. A. Asimptotika lyubogo poryadka sobstvennyh znachenij i sobstvennyh funkcij kraevoj zadachi SHturma—Liuvillya na otrezke s summiruemym potencialom [Asymptotics of an arbitrary order of eigenvalues and eigenfunctions of the Sturm-Liouville boundary value problem on an interval with summable potential] / V. A. Vinokurov, V. A. Sadovnichii // Izvestiya RAN. Ser .: Math. — 2000. — V. 64. — No. 4. — P. 47-108. [in Russian]
- Mitrokhin S. I. Asimptotika sobstvennyh znachenij differencial'nogo operatora chetvyortogo poryadka s summiruemymi koefficientami [The asymptotics of eigenvalues of the differential operator of the fourth order with summable coefficients] / S. I. Mitrokhin // Bulletin of Moscow University. Ser.: math., mech. — 2009. — No. 3. — P. 14-17. [in Russian]
- Savchuk A. M. Operatory SHturma-Liuvillya s singulyarnymi potencialami [The Sturm-Liouville operators with singular potentials] / A. M. Savchuk, A. A. Shkalikov // Mathematical notes. — 1999. — V. 66. — No. 6. — P. 897-912. [in Russian]
- Mitrokhin S. I. O spektral'nyh svojstvah odnogo differencial'nogo operatora s summiruemymi koefficientami s zapazdyvayushchim argumentom [On the spectral properties of a differential operator with summable coefficients with retarded argument] / S. I. Mitrokhin // Ufa Mathematical Journal. — 2011. — V. 3. — No. 4. — P. 95-115. [in Russian]
- Savchuk A. M. Regulyarizovannyj sled pervogo poryadka operatora SHturma-Liuvillya s –potencialom [Regularized trace of the first order of the Sturm-Liouville operator with –potential] / A. M. Savchuk // Uspekhi Math. — 2000. — V. 55. — №6 (336). — P. 155-156. [in Russian]
- Mitrokhin S. I. Ob izuchenii spektra mnogotochechnoj kraevoj zadachi dlya differencial'nogo operatora nechyotnogo poryadka s summiruemym potencialom [On the study of the spectrum of a multipoint boundary value problems for a differential operator of odd order with summable potential] / S. I. Mitrokhin // Matematicheskie zametki SVFU [Mathematical notes of North-Eastern Federal University]. — 2017. — V. 24. — No. 1. — P. 26-42. [in Russian]
- Naimark M. A. Linejnye differencial'nye operatory [Linear differential operators]. / M. A. Naimark // M.: Science. — 1969. — 528 p. [in Russian]
- Bellman. Differencial'no-raznostnye uravneniya [Differential-difference equations]. / R. Bellman, K. L. Kuk // M .: Mir. 1967. — 548 p. [in Russian]
