ОПРЕДЕЛЕНИЕ УСЛОВИЙ БЕЗОПАСНОГО ХРАНЕНИЯ И ТРАНСПОРТИРОВКИ НЕФТЕПРОДУКТОВ В ЕМКОСТЯХ РАЗЛИЧНОЙ ФОРМЫ

Шариков Ю.В. ¹, Снегирев Н.В.²

¹Профессор, доктор технических наук, ²студент, Национальный минерально-сырьевой университет «ГОРНЫЙ» (Горный университет)

ОПРЕДЕЛЕНИЕ УСЛОВИЙ БЕЗОПАСНОГО ХРАНЕНИЯ И ТРАНСПОРТИРОВКИ НЕФТЕПРОДУКТОВ В ЕМКОСТЯХ РАЗЛИЧНОЙ ФОРМЫ

Аннотация

В статье рассматривается применение программного пакета ConvEx при проектировании емкостей для хранения нефтепродуктов, склонных к саморазложению. Было исследовано влияние геометрической формы сосуда на критическую температуру хранения с целью повышения промышленной безопасности на нефтехранилищах

Ключевые слова: тепловой взрыв, безопасность, саморазложение, математическое моделирование.

Sharikov I.V. ¹, Snegirev N.V. ²

¹Professor, Dr. of Science (Chem. Engng.), ²student, National mineral resources university (mining university)

FINDING THE CONDITIONS OF HYDROCARBONS SAFE STORING AND TRANSPORTATION IN TANKS OF DIFFERENT SHAPES

Abstract

The article examines using the ConvEx software for designing of tanks for self-decomposition hydrocarbons storing. There was studied an influence of tank geometrical shapes for critical temperature of storing in order to increase an industrial safety of storages.

Keywords: thermal explosion, safety, self-decomposition, mathematical modeling.

Introduction

The current state of society is characterized by the increasing influence of the role of human technological activity on life environment. It is associated with increasing frequency of accidents. As the analysis of the causes and consequences of disasters, they are most often associated with working with hazardous chemicals substance. Runaway process occurrence involve to a thermal explosion [1].

At tank process analysis we must to consider a natural convection due to the temperature difference occurring at various points of space during storage and transportation of  low viscosity liquids

There are several approaches to solving this problem, beginning only the experimental determination of the critical conditions and ending with a numerical simulation based on the general very complex mathematical models. The power of modern computers and proven mathematical models, which can describe a complex multi-step reaction, allow to wide use a numerical simulation to study the convective processes in the liquid phase. ConvEx is a software suite allow to make these calculations [2].

Mathematical model, which adequately describes the natural convection in liquid multi-component reactive environment, uses the following assumptions:

• fluid is a Newtonian fluid;
• energy scattering due to viscous dissipation equal to nothing;
• thermodiffusion and diffusion thermal conductivity equal to nothing;
• uses Boussinesq approximation.

The Boussinesq approximation consist of the following:

• density depends on temperature and concentration, but is independent of pressure;
• change in density can be neglected everywhere except the members responsible for the lift force in the equation of momentum transfer.

Today there is no doubt that the model based on this approximations, adequately describes the natural convective processes in liquids. The mathematical model for:

• vertical cylinder is:

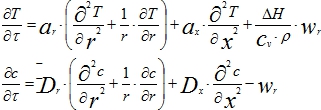
• infinite horizontal cylinder:

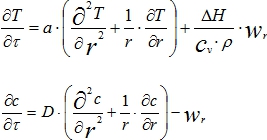
However, during model using to simulate the explosive reaction mode must be aware that the model becomes not adequate for new physical occurrences (for example, such as boiling), causes by the temperature increasing. So on the basis of calculations using this model we can conclude only that the thermal explosion occurs or not, but doesn’t analyze the process of thermal explosion evaluation. This is consistent with the main task of process safety: to prevent uncontrolled self-acceleration of reaction [3,4]. On practice the conclusion about the start of the thermal explosion can be made when a maximum temperature on the graph express bend upward.

The compound includes the following components:

• 0,7 mass. % of cumene hydroperoxide;
• 0,2 mass % of cumene (isopropylbenzol);
• 0,1 mass % of hydrogen oxide.

The critical diameter of tank is selected from the following causes: thermal explosion mustn’t occur earlier than after 50 hours under the worst storage conditions. The worst storage conditions is the temperature to which may become hot temperature of the tank wall in direct sunlight (assumed equal to 80^оС) in place of the thinnest tank insulation (assumed equal to 7 mm.). Rigid polyurethane foam is a heat insulation of both tanks.

The construction of vertical cylinder presents on fig. 1.

 

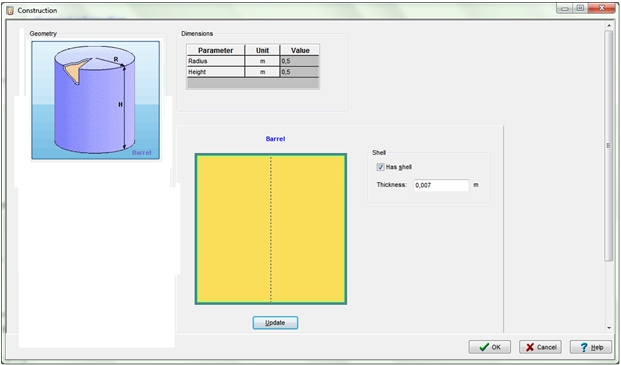
Fig. 1 - The construction of vertical tank

 

Further sets tank materials, heat insulation of tank, all the products involved and produced in the reaction and the boundary conditions. Fig. 2 and 3 show an example of setting the boundary properties and insulation properties (rigid polyurethane foam properties took from GOST 30732-2006).

 

Fig. 2 - Boundary conditions

 

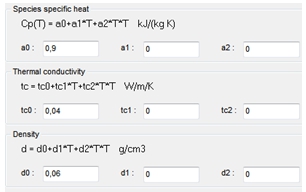
Fig. 3 - Properties of heat insulation

  Kinetic equations describing the processes in tank are shown in fig. 4  

Fig. 4 – Kinetics

  Conditions of the occurrence of thermal explosion is conveniently characterized by using the Frank-Kamenetsky’s parameter: Where: Q – energy-releasing effect of reaction, J; ρ – density of mixture, kg/m³; E – activation energy, J/mol; λ – thermal conductivity coefficient, ; R – universal gas constant, ; T – initial temperature of storage, K; r – radius of tank, m; k₀ – preexponential factor_.

If δ > δ_c, thermal explosion are expands, where for vertical tank with only conductive heat transfer conditions δc = 1. But in our case there is intense natural convection because of low viscosity of liquids, and natural convection influence on the tank processes characterized Rayleigh number:

Where: g – acceleration of gravity, m/s²; β_T – thermal volume-expansion coefficient, ; ν – kinematical viscosity, m²/s; a - thermal diffusivity, m²/s. Thermal diffusivity is calculated as:

Where c_p – specific heat, .

At Rayleigh numbers about 10³, the influence of convection on heat transfer becomes significant. It turns out that convection can delay the occurrence of thermal explosion. It means the critical value of the Frank-Kamenetsky in a convection exceed obtained considering only conductive heat transfer. In addition, natural convection increases the induction period of thermal explosion.

Experimental results and discussion

As a result of experiments with model critical diameter of vertical tank is D_crit = 1 m with H = 0,5 m.

By trial and error was found that the critical temperature for vertical cylinder equal to 58^оС. It means T_crit = 58^оС – is the temperature at which still no thermal explosion. Changing the temperature during the process shown in fig. 5.

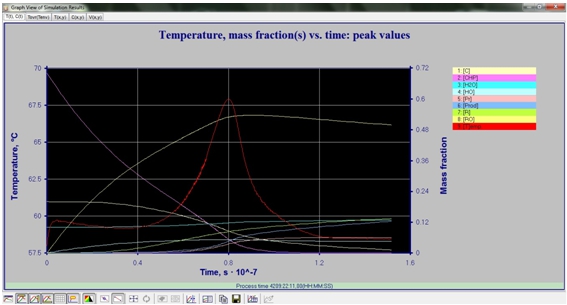
Fig. 5 - Changing of temperature during storage in vertical tank

 

Safe storage temperature is a temperature which below the critical on 10%, so

 Т_safe = 58 – 0,1·58 ≈ 52 ^оС

Frank-Kamenetsky’s parameter and Rayleigh’s coefficient calculated using MathCAD software in dimensions of SI units:

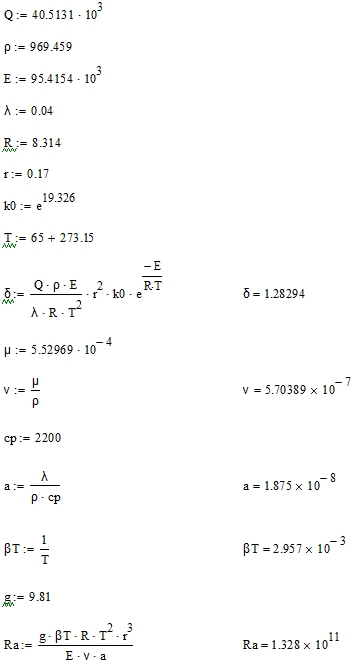
Conclusion

Horizontal cylindrical container has a slightly higher critical temperature storage (65 ° C vs. 58 ° C) but at a much smaller diameter (~ 3-fold) and the length substantially exceeding its diameter. This can lead to additional inconveniences during transport of such containers because of their length with a small capacity, which makes it less effective to use. Vertical cylindrical vessel possesses little lower critical temperature of the storage has a lower surface area, which makes it more advantageous in terms of heat transfer and therefore advantageous to store such a liquid mixture.

The foregoing procedure can be used to determine safe conditions for storage and use of potentially hazardous liquids. As it is evident from the foregoing for methods addressed using there is necessary a data of thermal decomposition kinetic, thermal-physical properties of research liquids, constructions materials and heat insulation materials.

Список литературы / References

1. Main dangerous of chemical industries, 1989, 671 p.
2. Kossoy A., Sheinman I., Evaluating thermal explosion hazard by using kinetics-based simulation approach, Process Safety and Envir. Protection. Trans IchemE, V. 82, Issue B6, November 2004, p.421-430. (B6 Special Issue: Risk Management)
3. Beloglazov I. N., Sharikov Y.V. Modeling of system. Part 1. Models synthesis of technological objects based on hydrodynamic equations and chemical kinetics. Saint – Petersburg, 2011, 108 p.
4. Beloglazov I. N., Sharikov Y.V. Modeling of system. Part 2. Methodical of numeric implementing. Saint – Petersburg, 2011, 118 p.