О КВАЗИРЕЛЯТИВИСТСКИХ УРАВНЕНИЯХ ДЛЯ ПЛАЗМЫ СОЛНЕЧНОЙ КОРОНЫ И ВАРИАНТ ОБЪЯСНЕНИЯ ЕЕ ВЫСОКОЙ ТЕМПЕРАТУРЫ
О КВАЗИРЕЛЯТИВИСТСКИХ УРАВНЕНИЯХ ДЛЯ ПЛАЗМЫ СОЛНЕЧНОЙ КОРОНЫ И ВАРИАНТ ОБЪЯСНЕНИЯ ЕЕ ВЫСОКОЙ ТЕМПЕРАТУРЫ
Научная статья
Трощиев Ю. В.*
ORCID: 0000-0001-6805-7001,
Московский государственный университет им. М.В. Ломоносова, Москва, Россия
* Корреспондирующий автор (yuvt@yandex.ru)
АннотацияВ статье выводятся квазирелятивистские уравнения из нерялитивистских внесением в них соответствующих изменений. Они включают в себя уравнения непрерывности, законы сохранения импульсов и энергий как для протонов, так и для электронов. Это необходимо, так как гидродинамические и электрические силы, действующие на них, примерно одинаковы, а, следовательно, их импульсы тоже примерно одинаковы. Обычная скорость протонов в солнечной короне порядка 500 км/с, а соответствующая скорость электронов для такого импульса составляет 0.953 c, поэтому электроны необходимо учитывать и они релятивистские. Выражения для столкновительных членов тоже адаптированы к релятивистским скоростям. Тепло, выделяющееся в результате трения между электронными и протонными потоками, оказывается достаточным для нагрева плазмы. Концентрация ne =1010 см-3 и количество выделяющейся при рассеянии энергии компенсируют большое время свободного пробега.
Ключевые слова: плазма, солнечная корона, релятивистские эффекты.
ON THE QUASIRELATIVISTIC EQUATIONS FOR THE SOLAR CORONA PLASMA AND A VARIANT OF RATIONALE OF ITS HIGH TEMPERATURE
Research article
Troshchiev Yu. V.*
ORCID: 0000-0001-6805-7001,
Moscow State University, Moscow, Russia
* Corresponding author (yuvt@yandex.ru)
AbstractThe quasi-relativistic equations are derived from nonrelativistic equations bringing amendments in them. They comprise of the continuity equations, and the conservation laws for impulses and energies both for protons and for electrons. It is so because the hydrodynamic and electric forces acting on them are similar, so, their impulses are also similar. The rife proton velocity in solar corona is 500 km/s and the consistent electron velocity for such impulse is 0.953 c, hence, the electrons must be taken into account and they are relativistic. Expressions for the collisional terms are also adapted to relativistic velocities. Heat emitting to warm protons due to friction between electron and proton flows is sufficient to heat plasma. Concentration ne = 1010 cm-3 and amount of scattering energy compensate large free path time.
Keywords: plasma, solar corona, relativistic effects.
IntroductionIt is substantial to decide correctly what processes in the solar corona must be investigated and what processes can be neglected. Relativistic equations of solar corona plasma are derived in this Research article from the classic equations [1]. Influence of the relativistic electrons at plasma heating is investigated. Electrons are often neglected in plasma because of the supposition of plasma neutrality. Moreover, solar corona plasma is often treated as collisionless (collisions in plasma are not bumping but veering over interaction). Nonetheless, electrons suffer collisions and it plays a role in plasma heating. The problems of solar corona plasma heating are being intensively investigated now, for example. But the problem of high temperature 106 K of the solar corona is still considered as unsolved. Some nonstandard source of extremely high temperature is proposed and investigated in this Research article. The result was reported by me at the conference [12].
Compulsory Consideration of Relativistic Effects while Modeling of the Solar Corona
If plasma is neutral then protons and electrons are settled equally. Thus, gradients of proton and electron pressures are similar and their impulses induced by pressure during a small time period dt are also similar.
Usual velocities of gas flows are 500 km/s in the quiet solar corona. If protons move at Vp=500 km/s then the classic equation for impulses mpVp=meVe gives superlight velocity Ve for electrons. mp, me – masses of proton and electron correspondingly. The relativistic equation for impulses
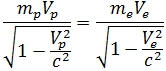 (1)
(1)
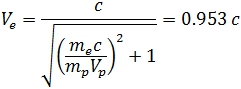 (2)
(2)
and increasing of their orthogonal mass in 3.3 times. Here c is light velocity. If the proton wind is 10 times stronger then electrons velocity is 0.9995 c and orthogonal mass increases in 31.5 times.
This is for gas dynamic forces. Let now plasma is diverted a little from neutrality. Then electric forces will bring the same impulses to protons and electrons during a small time dt because charges of proton and electron are the same. Magnetic forces change only the direction of impulses. So, we can suppose that the normal situation is moving of protons and electrons with approximately same impulses. This abstract reasoning leads to infer: 1) electrons must be taken into account in spite of their little mass because they carry similar to protons impulses, 2) if the devices register proton velocities 500 km/s in the solar corona then the electrons move there at relativistic velocities. The mechanisms of electron acceleration can be diverse. It seems to be an antinomy that protons and electrons move with different velocities in neutral plasma but this antinomy is resolved in the last section of this Research article that concerns the results of investigation.
The derived hereinafter relativistic equations are more precious than classical ones but they are derived from them, so, it is more natural to call the derived equations quasirelativistic.
Quasirelativistic Equations of Completely Ionized Plasma
It is a way of constructing of the equations in what follows. Some terms in section 4 are given for two-dimensional case without loss of generality. It is possible to write three-dimensional terms and include additional physical processes. The quasirelativistic system of equations includes the terms similar to the classical system in [1] with special corrections for relativistic case and for solving of the problem of high temperature of the solar corona. The corrections are formulation of impulse and energy conservation laws directly for impulses and energies and not for velocities and temperatures, adding one more term and rearranging of one term from the heat flows to the heat sources. The derivation of the friction term and of the corresponding heat source is made in an unusual manner to allow hydrodynamic velocities to be much greater than thermal velocities.
Quasirelativistic equations of completely ionized plasma are the following:
 (3)
(3)
where r = (x,y,z) – right-handed coordinates, t – time, indices ‘e’, ‘i’denote electrons and ions correspondingly; na – concentrations, – average impulses of the pResearch articles, ![]() – average heat energies of the pResearch articles, Va – velocities, ma – masses, pa – pressures, e – electron charge, Zi – ion charge in electron charge units, E – intensity of electric field, B – induction of magnetic field, Ffr – includes friction force between proton and electron flows and thermal force (Ffr is interaction between electrons and protons, so, its directions are opposite), qa – heat flows, Q – heat sources. Viscosity is neglected. PResearch article derivative is
– average heat energies of the pResearch articles, Va – velocities, ma – masses, pa – pressures, e – electron charge, Zi – ion charge in electron charge units, E – intensity of electric field, B – induction of magnetic field, Ffr – includes friction force between proton and electron flows and thermal force (Ffr is interaction between electrons and protons, so, its directions are opposite), qa – heat flows, Q – heat sources. Viscosity is neglected. PResearch article derivative is
![]() (4)
(4)
Symbolic index a can assume values ‘e’ or ‘i’. SI system of units is applied in (3).
The equations (3) are three-dimensional. It is more convenient to investigate two-dimensional equations in many cases. Reflecting reality they spend much less computer time and memory for calculations.
Two-dimensional equations look alike (3) with another sense of variables. Namely, Bx = By = 0, Bz = B and no variable depends on z coordinate. Components Ez = 0, Bx and By will remain zeros under these assumptions.
The more easy two-dimensional case is additionally Vez = Viz ≡ 0. z-components of friction and heat flows are linear combinations of the vectors u=Ve−Vi, ∇Te and ∇Ti, so, Fz = 0 and qez = qiz = 0 under zero z-velocities. It is important that they will remain zeros under relativistic summing of the velocities in this case.
Physical Values Transformations for Different Coordinate Systems
It is more convenient to calculate some physical values in the fixed coordinate system and some ones in the systems moving with the flows of protons or electrons. Thus, two relativistic orthogonal masses are introduced: mass in the fixed system for electron-proton collisions
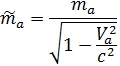 (5)
(5)
 (6)
(6)
where R – universal gas constant, µ – molar mass. One wave over a symbol denotes the fixed system. It can be omitted if there is no ambiguity. Two waves denote moving system.
Free path times of the pResearch articles (5) and (6) are different:
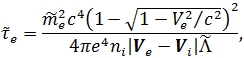 (7)
(7)
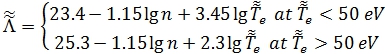 (8)
(8)
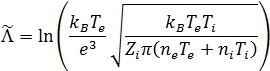 and
and
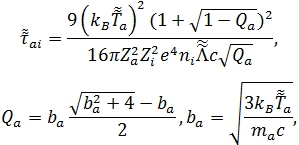 (9)
(9)
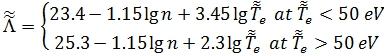 (10)
(10)
Gauss units system is conventional in astrophysics, so, it is used here and in what follows. Additionally, temperature in electron-volts is remained in practical formulas from [1] (e.g., (10)). Λ – Coulomb logarithm, kB – Boltzmann constant, Ze=1.
The fact that even at relativistic velocities electrons are at least 10 times lighter and move faster than protons is used in (7) and (9).
Let the set of three vectors e1 = Va/|Va|, e2, e3 in the fixed coordinate system is a right-hand coordinates. They are column-vectors in coordinate notation. Then the matrix A = (e1,e2,e3)T is a matrix of transformation of the fixed coordinate system to coordinates defined by these orts. The matrix AT = A−1 is a reverse transformation. The relativistic transformation of the velocity ![]() to the velocity
to the velocity ![]() is defined now
is defined now
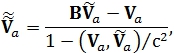 (11)
(11)
B=A−1DA, ![]() . diag denotes a diagonal matrix. The matrix B does not depend on the choice of e2 and e3. It can be represented independently from them:
. diag denotes a diagonal matrix. The matrix B does not depend on the choice of e2 and e3. It can be represented independently from them: ![]() , where E – unit matrix, P – projector to the linear span of e1.
, where E – unit matrix, P – projector to the linear span of e1.
The inverse transformation of the velocity is correspondingly:
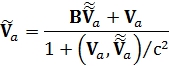 (12)
(12)
The rule of transformation of the force from the moving system to the fixed one follows from these formulas:
 (13)
(13)
Let now the average energy of the pResearch articles in the moving system is ![]() . Then their root mean square (rms) velocity is defined by the expression
. Then their root mean square (rms) velocity is defined by the expression
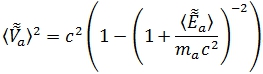 (14)
(14)
Let the square of the rms velocity in the fixed system be an averaging over the sphere of ![]() radius of the square of the velocity
radius of the square of the velocity ![]() :
:
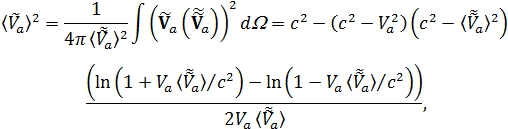 (15)
(15)
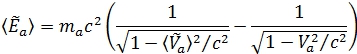 (16)
(16)
Time and volume also change while transition from one coordinate system to another but they compensate each other because of invariance of spatiotemporal intervals on Lorentz transform. So, the formulas (14)-(16) are transformations of heat energy for transitions from one coordinate system to another.
Physical Terms Included in the Equations
It is possible now to write expressions for friction, heat flows and sources. Friction consists of two components – friction force and thermal force:
![]() (17)
(17)
![]() (18)
(18)
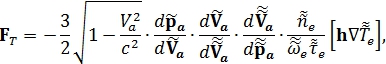 (19)
(19)
where ![]() – cyclotron frequency in the coordinate system moving with the flow, h – unit vector of magnetic induction direction.
– cyclotron frequency in the coordinate system moving with the flow, h – unit vector of magnetic induction direction.
The source being generated by electron heat flow consists of two components:
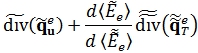 (20)
(20)
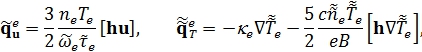 (21)
(21)
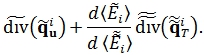 (22)
where
(22)
where 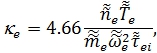 (23)
(23)
Usage of expressions from [1] bringing relativistic effects to them and additionally inclusion of the term ![]() gives:
gives:
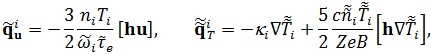 (24)
(24)
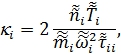 (25)
(25)
where ωi – cyclotron frequencies: ![]() . Another way to find is given hereinafter.
. Another way to find is given hereinafter.
The flows (21) and (24) are written in supposition of great values of cyclotron frequencies. Crux of the matter is that they define heat emitting due to electron and ion collisions.
It follows from (18) that force of friction is
![]() (26)
(26)
The kinetic energy is ![]() . Heat dW emitting in the unit value during dt due to friction is apparently
. Heat dW emitting in the unit value during dt due to friction is apparently
![]() (27)
(27)
so,
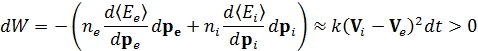 (28)
(28)
Electrons scatter more than protons and Ve>>Vi (it was assumed in expression for Fflow too). If all electron kinetic energy transforms to heat in collisions then
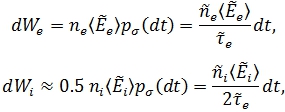 (29)
(29)
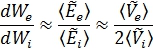 (30)
since
(30)
since
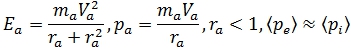 (31)
where
(31)
where These formulas define the minimal estimation for heating of proton gas. To find the upper estimation it is natural to assume dWe = dWi = 0.5dW.
Results of Investigation
Temperature 10.27·106 K corresponds to proton velocity 500 km/s and temperature 8.99·109 K corresponds to electron velocity 0.953 c. So, the high temperature source for plasma heating exists in spite of solar surface temperature 6000 K. This mechanism is transformation of hydrodynamic velocities into heat velocities in electron-proton collisions. Estimation of energy coming and losing is another physical problem which is out of the framework of this Research article. But it worth to note that Sun produces much more energy than it is necessary to heat the corona and that solar corona radiation is very faint.
The lower estimations of temperature increasing depending on proton velocity at concentration 1010 are in the Table 1 according to the formula (32).
Table 1 – Rate of temperature increasing (K/s) depending on velocity of protons (km/s)
| Vp | 10 | 100 | 500 | 1000 |
| dT/dt | 20000 | 16000 | 110 | 39 |
Preliminary computer calculations of the system (3) by difference schemes corroborated increasing of temperature. The initial pressure gradients cause then plasma oscillations that seem to be the main mechanism of electron-proton collisions. Langmuir frequency is approximately 106 s−1 here. These oscillations also answer the question how it is possible for protons and electrons to move with different velocities in neutral plasma. And the calculations confirm similarity of their pulses.
| Конфликт интересов Не указан. | Conflict of Interest None declared. |
Список литературы / References
- Braginskii S. I. Transport processes in a Plasma / S. I. Braginskii // Reviews of Plasma Physics, ed. M. A. Leontovich, Consultants Bureau – 1965 – V. 1 – P. 205.
- Загадки солнечной короны // Наука в России – 2011 – № 4 – С. 61-63.
- Molotkov I.A. Solar corona top heating / I.A. Molotkov, N.A. Ryabova // Geomagn. Aeron – 2016 – V. 56 – № 3 – P. 264-267. doi: 10.1134/S0016793216030130
- Badalyan O.G. To the problem of solar coronal heating / O.G. Badalyan, V.N. Obridko // Astron. Lett. – 2007 – V. 33 – N 3 – P. 182-191. https://doi.org/10.1134/S106377370703005X
- Somov B.V. Plasma astrophysics. Part II. Reconnection and flares / B.V. Somov // Springer – 2006 – 417 P.
- Kovalev V.A. The Role of Collisions in the PResearch article Acceleration in Solar-Flare Magnetic Traps / V.A. Kovalev, B.V. Somov // Astron. Lett. – 2003 – V. 29 – N 6 – P. 409-415. doi: 1134/1.1579790
- Bilenko I.A. On the regimes of heating during solar flares / I.A. Bilenko, V.A. Kovalev // Astron. Lett. – 2009 – V. 35 – N 11 – P. 791-797. doi: 10.1134/S1063773709110061
- Kovalev V.A. The acceleration of evolution caused by a decrease of dissipation / V.A. Kovalev // The complex systems – 2017 – N 1(4) – P. 13-20.
- Bret A. Collisional behaviors of astrophysical collisionless plasmas / A. Bret // J. Plasma Phys. – 2015 – V. 81. doi:10.1017/S0022377815000173
- Kurkina E. S. Formation of Thermal Structures with Blowup During Solar Flares / E. S. Kurkina, E. D. Kuretova, V. A. Kovalev // Comput. Math. Model. –2015 – V. 26 – P. 144-155. doi:10.1007/s10598-015-9262-5
- Kurkina E. S. Small-Scale Heat Localization with Blowup in the Magnetic-Tube Cross Section During a Solar Flare / E. S. Kurkina, Y. V. Troshchiev, V. A. Kovalev, et al // Comput. Math. Model. – 2016 – V. 27 – P. 395-416. doi:10.1007/s10598016-9330-5
- Troshchiev Y.V. Quasirelativistic equations for the solar corona plasma and a variant of rationale of its high temperature / Y.V. Troshchiev // Theses of the conference High Enegry Phenomena in Relativistic Outflows VI, Moscow, Russia, IKI RAS, 11-15 September – 2017 – P. 22.
Список литературы на английском языке / References in English
- Braginskii S. I. Transport processes in a Plasma / S. I. Braginskii // Reviews of Plasma Physics, ed. M. A. Leontovich, Consultants Bureau – 1965 – V. 1 – P. 205.
- Zagadki solnechnoi korony [Mysteries of the solar corona] // Nauka v Rossii [Science in Russia] – 2011 – № 4 – P. 61-63. [in Russian]
- Molotkov I.A. Solar corona top heating / I.A. Molotkov, N.A. Ryabova // Geomagn. Aeron – 2016 – V. 56 – № 3 – P. 264-267. doi: 10.1134/S0016793216030130
- Badalyan O.G. To the problem of solar coronal heating / O.G. Badalyan, V.N. Obridko // Astron. Lett. – 2007 – V. 33 – N 3 – P. 182-191. https://doi.org/10.1134/S106377370703005X
- Somov B.V. Plasma astrophysics. Part II. Reconnection and flares / B.V. Somov // Springer – 2006 – 417 P.
- Kovalev V.A. The Role of Collisions in the PResearch article Acceleration in Solar-Flare Magnetic Traps / V.A. Kovalev, B.V. Somov // Astron. Lett. – 2003 – V. 29 – N 6 – P. 409-415. doi: 1134/1.1579790
- Bilenko I.A. On the regimes of heating during solar flares / I.A. Bilenko, V.A. Kovalev // Astron. Lett. – 2009 – V. 35 – N 11 – P. 791-797. doi: 10.1134/S1063773709110061
- Kovalev V.A. The acceleration of evolution caused by a decrease of dissipation / V.A. Kovalev // The complex systems – 2017 – N 1(4) – P. 13-20.
- Bret A. Collisional behaviors of astrophysical collisionless plasmas / A. Bret // J. Plasma Phys. – 2015 – V. 81. doi:10.1017/S0022377815000173
- Kurkina E. S. Formation of Thermal Structures with Blowup During Solar Flares / E. S. Kurkina, E. D. Kuretova, V. A. Kovalev // Comput. Math. Model. –2015 – V. 26 – P. 144-155. doi:10.1007/s10598-015-9262-5
- Kurkina E. S. Small-Scale Heat Localization with Blowup in the Magnetic-Tube Cross Section During a Solar Flare / E. S. Kurkina, Y. V. Troshchiev, V. A. Kovalev and others // Comput. Math. Model. – 2016 – V. 27 – P. 395-416. doi:10.1007/s10598016-9330-5
- Troshchiev Y.V. Quasirelativistic equations for the solar corona plasma and a variant of rationale of its high temperature / Y.V. Troshchiev // Theses of the conference High Enegry Phenomena in Relativistic Outflows VI, Moscow, Russia, IKI RAS, 11-15 September – 2017 – P. 22.
