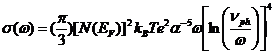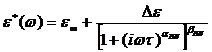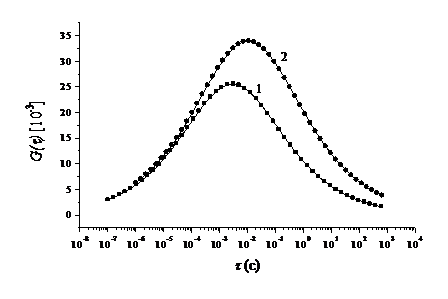НИЗКОЧАСТОТНАЯ ДИЭЛЕКТРИЧЕСКАЯ РЕЛАКСАЦИЯ В СЛОЯХ СТЕАРАТА СЕРЕБРА

Горяев М.А. ¹, Кастро Р.А.², Смирнов А.П.³

¹Доктор технических наук, ²Доктор физико-математических наук, Российский государственный педагогический Университет им. А.И.Герцена

НИЗКОЧАСТОТНАЯ ДИЭЛЕКТРИЧЕСКАЯ РЕЛАКСАЦИЯ В СЛОЯХ СТЕАРАТА СЕРЕБРА

Аннотация

Исследована низкочастотная диэлектрическая релаксация в тонких слоях стеарата серебра. Рост диэлектрической проницаемости с уменьшением частоты, а также с ростом температуры связан с наличием в исследуемом материале дипольно-релаксационного механизма. Дисперсия фактора диэлектрических потерь связана, вероятно, с вкладом механизма релаксационных потерь и проводимости. Из диаграммы Коула-Коула можно сделать вывод о том, что стеарат серебра является недебаевским диэлектрическим материалом с широким распределением релаксаторов по временам релаксации согласно модели Коула-Коула.

Ключевые слова: стеарат серебра, диэлектрическая спектроскопия, диэлектрическая релаксация.

 

Goryaev M. A.¹, Castro R. A.², Smirnov A. P.³

¹ PhD in Engineering, ² PhD in Physics and Mathematics, Herzen State Pedagogical University of Russia, St. Petersburg

LOW-FREQUENCY DIELECTRIC RELAXATION IN SILVER STEARATE LAYERS

Abstract

The low-frequency dielectric relaxation process in silver stearate layers was studied. The increasing of dielectric permittivity with frequency decreasing and temperature increasing in studied sample are associated with the dipole-relaxation polarization mechanisms. The dispersion of loss factor could be connected with the contribution of relaxation mechanism and conductivity. The shape of the Cole-Cole diagram shows that silver stearate is a non-Debye dielectric material characterized by a wide distribution of relaxators, according to the Cole-Cole relaxation model.

Keywords: silver stearate, dielectric spectroscopy, dielectric relaxation.

 

Introduction

Photothermographic materials based on compositions of silver halides and of fatty acids salts, in particular, silver stearate, are widely applied for efficient registration of optical images [1]. During the manufacturing of the photosensitive composition, silver halide is synthesized on the surface of the particles of fatty acids salts (silver stearate) [2, 3]. Spectral sensitization of photothermographic materials is performed by using different dye-sensitizers introduced into such heat-developable composition. Consequently spectral sensitization is possible in a wide optical band (from blue to near-infrared) [3]. Silver stearate participates in the processes of sensitization and latent image development. [3-5]. Therefore, the study of electrical and optical properties, as well as the identification of structural features of this material represents an actual task. The objective of this work was the investigation specific features of dielectric relaxation and charge transport processes in the silver stearate layers and this relationship to structural features of the material. It is known that structural features of the disorder systems are caused by existence of the defect electronic or impurity centers [6, 7]. The assessment of influence of such centers on electrophysical properties of materials allows to reveal the nature of the processes proceeding in them and to broaden areas of their practical application.

Experimental details

Silver stearate is produced by the exchange reaction of sodium substitution under the excess concentration of silver nitrate particles of about one micron. Thus, in electrophysical research monolithic samples of this substance are used, press-formed into a tablet shape. Films with thickness of 0.5 mm and diameter of 10 mm were used. Specific features of the dielectric relaxation processes were studied by the dielectric spectroscopy method with “Concept 81” system (Novocontrol Technologies). This method has been applied successfully in the recent studies of electronic processes in systems of varying structural disorder [8-10]. Spectra of the complex dielectric permittivity were calculated from the impedance data. The temperature and frequency dependences of dielectric parameters were measured in a wide frequency range (10^-2…10² Hz) and temperature band (273- 353 K). The operating voltage was 1.0 V. The error of measurements and calculations of physical parameters did not exceed 3%.

Results and discussion

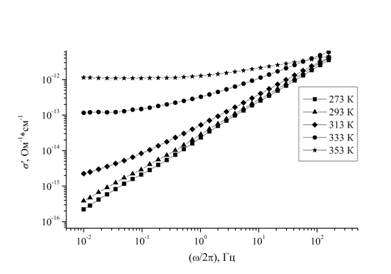
Figure 1 shows the frequency dependence of the real part of complex conductivity at different temperatures. The behavior pattern of this dependence reveals that the conductivity in silver stearate layers increases with frequency, by the law σ(ω)≈Аω^s, where A is a constant, ω – circular frequency, s – the exponent.

Figure 1. Frequency dependence of the silver stearate conductivity at different temperatures

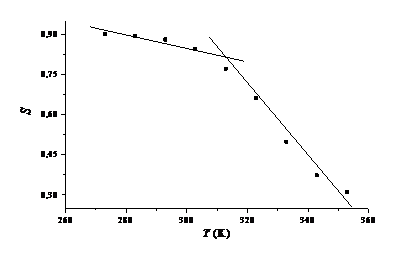
Figure 2 shows the temperature dependence of the exponent s, which takes values s = 0.30...0.90. This diagram has two areas, the weak (T=273...303 K) and the strong dependence (T=313...353 K). The observed patterns reveal of the possible hopping mechanism of conductivity in silver stearate layers.

According to the Austin-Mott model [11], the alternating current conductivity in disordered systems is determined by the electron jumps between the pairs of localized states at the Fermi level.

Figure 2. Temperature dependence of the exponent s

The model with random hopping energy [12] assumes that the elementary polarization act represents an over-barrier transition of one electron from one state to another and the activation energy of the center is determined by the Coulomb interaction. In this case, the value of the exponent s lies within the range 0.90-0.30 at room temperature and decreases with the increase in temperature. As is known, the conductivity is linked with the density of localized states N(E_F) at the Fermi level by the ratio:

                                                          (1)

where α is the attenuation parameter of wave functions of the localized states, ν_ph – the phononic frequency. By taking , , at frequency  and the temperature T=293K, we obtain the density value for the localized states at the Fermi level, .

According to the temperature dependence of the conductivity σ', we can conclude that the charge transport in the studied structures is a thermally activated process. We have also detected the exponential temperature dependence in σ', with two sites with activation energy Е_а1=0.10 eV and Е_а2=0.48 eV, respectively.

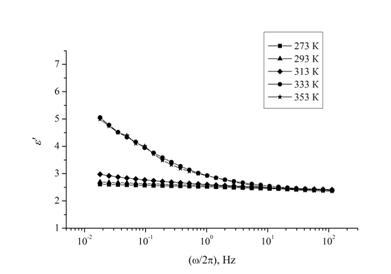
The frequency dependence of dielectric permittivity ε' is characterized by the presence of weak dispersion, which becomes more noticeable in the lower frequency range (fig. 3). Considering the structural features of silver stearate and the presence of quasi-dipole formations in the form of carboxyl tails there, we can conclude that the dipole-relaxation polarization represents the determining polarization mechanism in the studied frequency range [13]. The increase in dielectric permittivity constant along with the rising temperature can be explained by the connection of the dipole polarization mechanism with thermal motion of the molecules. Consequently, the dipoles are weakly oriented at low temperatures. In our case, a sharp increase in ε' values was observed with the increase in temperature, starting from T = 303 K.

Figure 3. Frequency dependence of dielectric permittivity ε' of silver stearate layers at different temperatures

The existence of a certain temperature value, which changes the nature of temperature and frequency dependences of conductivity and dielectric permittivity, indicates either the possible existence of a low-temperature phase transition, or the onset of the temperature area associated with the first high-temperature transition (at T=398 K [14]). This factor requires more research including structural methods for studying materials, such as DSC, AFM, SEM, etc.

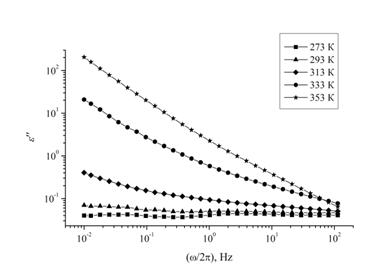
The observed dispersion of the loss factor ε" (fig. 4) is more likely associated with the manifestation of the relaxation loss mechanism. The increase in dielectric loss with temperature can be explained by the increasing contribution of conductivity losses.

In many dielectric materials relaxation processes are associated with the existence of a set of relaxation times, rather than a single relaxation time. In this case, we are dealing with the distribution of the relaxation time and, therefore, the activation energy. This distribution can be associated with the manifestation of relaxation processes of different nature or with the distribution of the dipole concentration in the structure. In the case of ion hopping processes, we assume that the potential energy is changed after each jump and some time is needed for getting round to the minimum potential energy. When the contribution of a large number of mobile defects is considered, we obtain a set of relaxation times [11].

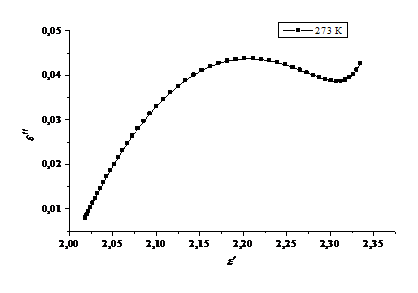
The deviation from the classical Debye model of relaxation for the case of single relaxation time can be studied using the Cole-Cole or the Cole-Davidson model representations for the cases of symmetric and asymmetric distribution of relaxation times, respectively. From Cole – Cole diagram (ε^’’=f(ε^’) dependence), the existence of the relaxation time distribution will be manifested by the deviation from hemispherical dependence with the radius of the hemisphere (ε_s-ε_∞)/2       (Fig. 5).

Figure 4. Frequency dependence of dielectric loss factor ε" in silver stearate layers at different temperatures

Figure 5. Cole-Cole diagram for silver stearate samples at temperature T=273 K.

The existence of the non-Debye relaxation mechanism is confirmed when estimating the values of parameters in the two-parameter Havriliak - Negami empirical function (HN) [13]. The results of measuring dielectric spectra were approximated using Winfit 3.3 software (Novocontrol Technologies GmbH)    (Fig. 6). Based on this approximation, locations of the dielectric loss maxima and the area of conductivity contribution were highlighted and the HN parameters for the studied polarization processes were determined, by the expression:

                                                                      (2)

where ε_∞ is the high-frequency limit of the real part of dielectric permittivity, Δε– the dielectric increment (the difference between the low-frequency and high-frequency limits), ω=2πf, α_HN and β_HN – shape parameters describing, respectively, the symmetric (β=1.00 – the Cole-Cole distribution) and asymmetric (α=1.00 – the Cole-Davidson distribution) expansion of the relaxation function. Taking into account the error of approximation, we can conclude that in the studied range of frequencies and temperatures in silver stearate layers, the Debye oscillation process is observed with the distribution of relaxation times, in concordance with the Cole-Cole model. The relaxation times distribution function G(τ) calculated for the studied silver stearate samples is shown in figure 7.

Figure 6. Approximation of the experimental dependence of the loss factor ε^’’ from frequency function (HN) for the temperature T=303 K.

Figure 7. Relaxation times distribution function G(τ) calculated for the studied silver stearate samples at two different temperatures, Т. 1 – 283 К, 2 – 303 К.

Conclusions

Frequency dependence of the real part of the conductivity has the form  that reveals the existence of a hopping mechanism of conduction in localized states. The temperature dependence σ is characterized by the presence of two areas with different activation energy.

The increase in dielectric permittivity along with decreasing frequency and increasing temperature, as well as the increase in the magnitude of dielectric losses with temperature are most likely associated with the manifestation of the dipole-relaxation polarization and conduction mechanisms. The shape of the Cole-Cole diagram shows that silver stearate is a non-Debye dielectric material characterized by a wide distribution of relaxators, according to the Cole-Cole relaxation model.

Acknowledgments

This research was supported by Ministry of Education and Science of the Russian Federation (project № 2014/376)

References

1. Morgan D.A. 1993 Phot. Sci. 41 108
2. Goryaev M. A. 1994 Russian Journal of Applied Chemistry 67 858
3. Goryaev M. 2013 Physical principles of photochemistry of the solid. Photolysis of inorganic solids (Saarbrucken: Lambert Acad. Publ.) (in Russian)
4. Goryaev M. A., Smirnov A. P. 2012 RSPU Proceedings 144 29 (in Russian)
5. Goryaev M. A. 1994 Soviet Technical Physics Letters 20 871
6. Castro R.A., Nemov S.A., Seregin P.P. 2006 Semiconductors 40 898
7. Anisimova N.I., Bordovsky G.A., Bordovsky V.A., Castro R.A. 2002 Radiation Effects and Defects in Solids 156 359
8. Castro R.A., Bordovsky G.A., Bordovsky V.A., Anisimova N.I. 2006 Journal of Non-Crystalline Solids 352 1560
9. Castro, R.A., Bordovsky, V.A., Grabko, G.I. 2009 Glass Physics and Chemistry 35 43
10. Afanasiev V.P., Vendik I.B., Vendig O.G., MedevedevaN.Yu, Odit M.A., Sitnikova M.F., Petrov A.A., Sokolova I.M., Chigirev D.A., Kastro R.A. 2012 Glass Physics and Chemistry 38 63
11. Austin J.G., Mott N.F. 1969 Phys. 18 41
12. Gutenev M. S. 1983. Physics and chemistry of glass 9 291(in Russian)
13. Kremer F, Schoenhals A 2003 Broadband dielectric spectroscopy (Berlin Heidelberg: Springer)
14. Goryaev M.A., Castro R.A., Smirnov A.P. 2014 Journal of Physics: Conference Series 572 012041
15. Castro R.A., Bordovsky V.A., Anisimova N.I., Grabko G.I. 2009 Semiconductors 43 365