ДИСБАЛАНС ЭНЕРГИЙ ЭЛЕКТРОННОГО ПУЧКА В ИНДУКЦИОННОМ ЦИКЛИЧЕСКОМ УСКОРИТЕЛЕ

Москалев В.А.¹, Сергеев Г.И.²

¹Доктор технических наук, профессор; ²Кандидат технических наук, старший преподаватель, Национальный исследовательский Томский политехнический университет

ДИСБАЛАНС ЭНЕРГИЙ ЭЛЕКТРОННОГО ПУЧКА В ИНДУКЦИОННОМ ЦИКЛИЧЕСКОМ УСКОРИТЕЛЕ

Аннотация

При индукционном циклическом ускорении токов заряженных частиц, превышающих сотни ампер, значительная доля энергии запасается в электромагнитном поле самого пучка. Это приводит к дисбалансу энергий, т.е. несоответствию между энергией, которую пучок получает от ускоряющего поля бетатрона, и энергией, необходимой пучку для устойчивого движения на равновесной орбите, что является одной из причин неустойчивости пучка и значительных потерь ускоряемого заряда.

Очевидно, полезно оценить степень возникающего дисбаланса и рассмотреть возможные меры по устранению его негативного воздействия на процесс ускорения. В статье проводится такая оценка.

Ключевые слова: Индукционный циклический ускоритель, килоамперные токи, неустойчивость циркулирующего пучка, дисбаланс энергий.

Moskalev V.A.¹, Sergeev G.I.²

¹DSc in Engineering, Professor; ²PhD in Engineering, Senior Lecturer, National Research Tomsk Polytechnic University

IMBALANCE OF ELECTRON-BEAM ENERGY IN INDUCTION CYCLIC ACCELERATOR

Abstract

Under inductive cyclic acceleration of charged particles the beam current of which exceeds several hundred amperes, a large amount of energy is accumulated in its electromagnetic field. These results in energy imbalance, i.e. inconsistency between energy received by the electron beam from betatron’s eddy electric field and energy required for the electron beam to stably move along the equilibrium orbit. This is one of the reasons for beam instability and large loss of accelerated particle charge. Thus, the paper presents an estimation of the occurred imbalance degree and considers possibilities of eliminating its negative effect on the process of acceleration.

Keywords: induction cyclic accelerator, beam-currents of thousands amperes, circulating-beam instability, energy imbalance.

In induction cyclic accelerators (betatrons) electron current is accelerated up to one ampere or so, while in pulsed air-cored betatrons and compressors it achieves hundreds amperes. In cyclic accelerating, the maximum limit of accelerated current is determined by the fact that electrons circulating within a closed orbit for a long time cause a resonant excitation of particle oscillations that results in a considerable amount of trapped charge getting the walls of the vacuum chamber and then dropping out of acceleration.

Induction method allows accelerating current by an order of magnitude. Late in the past century many efforts have been made to increase accelerated currents up to several kiloamperes. Test installations were designed to provide stability for circulating beam-currents using toroidal, stellarator fields, and their combination [1], [2]; installations for acceleration of strip electron beams [3], [4]. Also, research was carried out into a beam-focusing using a system of solenoids with reversed field direction [5]. nevertheless, no one of these installations allowed obtaining design parameters.

In accelerating electron charge equivalent to a circulating current of hundreds and thousands amperes, a significant loss of beam charge is observed in a betatron during its operating cycle.

The electron beam receives initial energy during injection, and its subsequent growth depends on eddy electric field of the induction accelerator. During acceleration, the electron beam partially loses its energy due to heating residual gas and vacuum chamber’s walls, emission of radio frequency, etc. Therefore, the total energy E of the beam at any given time is less than the amount of energy E_i of injected electrons and energy W received by the beam from the eddy electric field of the betatron to the given moment. The result is:

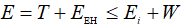              (1)

A kinetic energy of the electron beam is

T = (m − m₀) c² N  or T = E₀ (γ − 1) N,          (2)

where N is the number of circulating electrons; с is the speed of light; m₀ и m are mass at rest and relativistic mass of an electron respectively; E₀ is rest energy of an electron; γ is relativistic factor.

The electric field of the beam is concentrated in the space-limited volume of the vacuum chamber. A magnetic component of the beam energy is defined by the charge density and its movement speed. Thus, the electromagnetic field energy of a beam can be written as

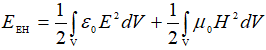            (3)

where Е and Н are electric and magnetic fields of the electron beam respectively; dV is the differential of volume. In case the electron beam density is uniform throughout its bulk and R₀ >> r₀ (R₀ is the radius of equilibrium orbit; r₀ is the radius of cross-section of a toroidal beam), we then obtain the electromagnetic field energy:

          (4)

where f (μ, r_p, r_с) is the function which takes into account magnetic characteristics of the magnetic circuit material μ; curvature r_с of conducting coating of the accelerating chamber, and the interval r_p between the median plane and the pole: 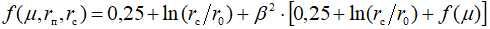             (5)

Energy of electrons injected into the chamber can be written as

             (6)

where U_i is the voltage of injection; N_i is the number of injected electrons; E_i = m_ic² is energy of electrons in injecting; E₀ is rest energy of an electron.

Energy W received by the beam from the eddy electric field during t_i  – t time interval can be expressed as

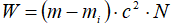             (7)

Taking into account (2) and (4) – (7), inequation (1) takes the form: 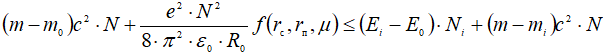        (8)

After simple rearrangements we obtain

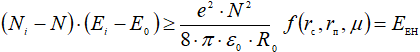       (9)

From (9), we get the electromagnetic field energy Е_ЕН of the electron beam circulating within the betatron which can be obtained in case

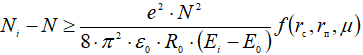        (10)

i.e. due to the appropriate number of electron lost during the process of acceleration. Thus, inequation (10) can be interpreted as electron loss stipulated by the energy imbalance. This loss grows proportionally to the square number of accelerated particles resulting in limitation of the accelerated charge value. This is essential in designing betatrons having beam-currents of several kiloamperes. Energy imbalance can be represented by a relation between the electromagnetic field energy E_EH and its kinetic energy T. Dependence between the energy imbalance and the acceleration time t can be obtained by assumption that the radius of cross-section of a toroidal beam is changed in accordance with the following law:

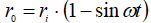         (11)

where r_i is the radius of the beam in the moment of injection.

Values of coefficient β (relativistic factor) in equation (5) can be detected at any time of acceleration using the known electron velocity:

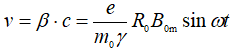     (12)

where B_0m is the amplitude of magnetic field induction on the radius of the equilibrium orbit R₀.

Since 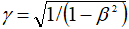, we obtain

Let , we get

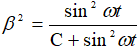            (13)

With respect to (11) and (13), we modify expression (4):

            (14)

while expression (2) can be written as

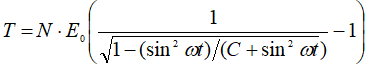          (15)

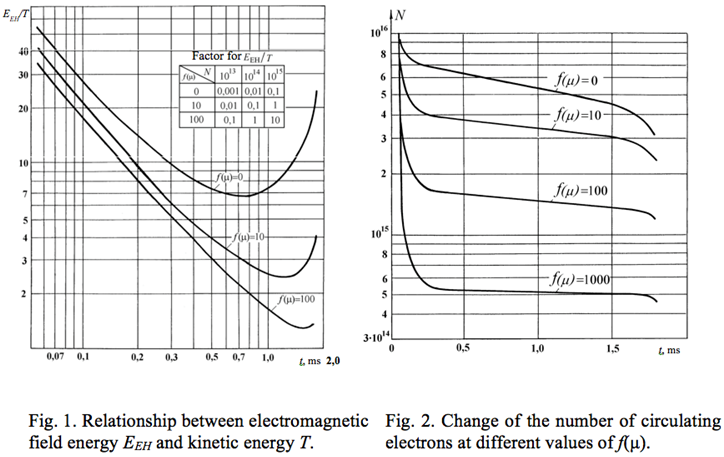
Relation E_EH / T is presented in Figure 1 at different values of f (μ) and N. With respect to E_EH / T, it is obvious that the larger the charge accelerated by a cyclic accelerator, the larger imbalance between energy required for a circulating-beam instability to move along the equilibrium orbit and energy received by the electron beam from  eddy electric field of betatron.

Substituting inequation for equation in (10), the upper boundary of a possible value N(t) can be found.

The results calculated for the number of circulating electrons allowing for f(μ) function are presented in Figure 2. This calculation is theoretical because, in fact, f(μ) function is also a function of time that has not been taken into account. As for the assumed law 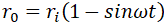 by which the radius of cross-section of a toroidal beam changes, it can be different. However, a type of the dependence between the particle loss and the time of acceleration is preserved (a rapid decrease at the beginning of the cycle and an increase at its end).

Figures 1 and 2 contain plots showing that owing to energy imbalance even minimum electron losses in high-energy betatrons are considerable, and they should not be neglected.

Loss of electrons caused by energy imbalance is proportional to the square number of accelerated particles in a beam, and this loss increases rapidly with the growth of accelerating charge.

From Figure 2 it follows that design of an air-cored betatron is advisable to minimize losses, at f (μ) = 0.

The loss of charged particles is inversely related to the radius of the equilibrium orbit R₀. However, the increase of its radius is irrational because it leads to the increase of accelerator’s weight ~ R₀³.

To eliminate a part of the known instabilities of the electron beam generated by cyclic accelerators, various systems of magnetic field correction and compensation systems of reflecting currents have been developed. These systems are rather technically complex, they do not compensate energy consumption for generation of electromagnetic field. They merely slightly lower the value of energy imbalance.

To eliminate electron loss due to the energy imbalance is possible to carry out by introducing the additional energy source into the betatron that should compensate energy required for a magnetic field generation (9). Thus, it is necessary to create the additional eddy electromagnetic force:

e_ad              (16)

Thus, the energy imbalance or inconsistency between energy received by the electron beam from betatron’s eddy electric field and energy required for the electron beam for its stable movement is equivalent to distortion of betatron relationship 2:1 and is one of the main causes of beam instability and significant loss of accelerating charge at current acceleration of several kiloamperes.